Mathematics in front of 2nd language

1991/10/01 Ansa, Lurdes - Elhuyar Edizioak Iturria: Elhuyar aldizkaria

• Matematika

Elhuyar: Where did you come to this topic?

J. F. F. L.:

It was not a topic that had been raised sharply; before being a professor at the University he taught mathematics at an Ikastola model B. They were high school students and the leap to Middle School was immediate. Although these students worked mathematics in Spanish, the intention of the teachers was to integrate the largest number of students in the D model of Middle Education, it was not illogical to think that they could have problems. This research began with the belief that it was urgent to verify, analyze, and classify this forecast.

PaP: What are the objectives of this work?

J. F. F. L.:

One of the main objectives proposed by us was to establish the scientific basis for rethinking teaching so that students of model B did not have problems working mathematics in Basque when they joined model D in the Middle Education. Before, however, it was necessary to analyze whether or not they had problems, since it could have been possible that there was no problem and that ours was a mere suspicion.

The objective of model B is clear: Train so that any student of 8th year can follow any topic in both Basque and Spanish, for which each center will put the necessary means. According to this theoretical approach, when going to the Middle School they would not have inconvenience either in Spanish or in Basque, but things almost never happen like this (and I refer to mathematics, which is our subject of study), among other things, because in some centers we work on the mathematics we study in Spanish.

The main objective of this thesis would be related to this concern: That is, whether or not the objective marked by model B is met, and how it affects students in case of non-compliance.

PaP: Why study the mathematical binomial/language?

J. F. F. L.:

Although it seems that mathematics and language are distanced, in any teaching process (including mathematics), the influence of language is undeniable. As Sánchez Carrion says in his book A future for our past, the teacher is teaching any topic, but he is always working the language. Therefore, to want to analyze the relations between mathematics and language is not so strange.

In bilingual contexts the problem is sharpened, since to be truly bilingual it is necessary to be able to use both languages in any topic, even in mathematics. Here real problems begin to emerge, since this is not fully met in model B.

The use of both languages, on the other hand, should in no case prevent the assimilation of the different matters, which no one would admit. In any program, whether monolingual or bilingual, the minimum objectives must be reached, even if each center has the freedom to choose methodology, material, resources, etc. If, in addition to achieving it, he mastered the 2nd language or the 3rd, better.

Although theoretically it was this way, we thought it was interesting to know what really happens and to this we set out; to analyze whether bilingual teaching is an obstacle to assimilation of mathematical contents.

PaP: To what extent can language condition the process of introduction of mathematics?

J. F. F. L.:

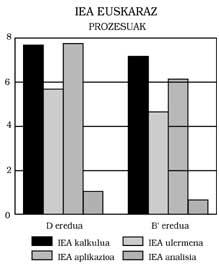
As mentioned above, language conditions the teaching of any subject, and mathematics is not left out of this general rule. So far we have talked about mathematics in its entirety, but, following Bloom's taxonomy, it is convenient to differentiate different processes such as calculation, understanding and application. In each of these languages it influences different. The importance of symbolic language in the calculation is: 3x4=12 is easily understandable in any language, of course, with minimal mathematical knowledge. Without missing too much, it can be thought that those of model B will not have too many problems in this process, but in the development of understanding, that is, in the understanding of the manifestations of the teacher or of a written text. In the application, that is, in the resolution of problems, the language is particularly important.

These last two processes will be the ones that we will have to analyze especially, since in them it influences mainly the language, and in our case, when speaking of language 2, not of the mother tongue, it seemed to us that there could be problems.

PaP: What is currently the B.O.P.V. and the organization of teaching mathematics in the U.B.I?

J. F. F. L.:

Three linguistic models are distinguished in the Basque Autonomous Community:

1. Model A, especially aimed at children erdaldunes. Everything is taught in Spanish and among other minimal objectives would be the learning of the Basque language; learning “euskera” and not “euskera”.
2. The model D, where everything is done in Basque, with Spanish as a subject.
3. Finally, we find the model B that we have studied, in which the Basque language and Spanish are treated theoretically at least at the same level, but work mathematics, writing and reading in Spanish, while the rest of subjects are taught in Basque. Why does this happen? In some theories and studies it has been shown that for there to be no delays in these important topics it is necessary to take the basic concepts in the mother tongue.

Although in the B.O.U. These three models are differentiated, the same does not happen in the Middle Education, since here there are only two models, the models A and D. In theory, students of model B. Once finished, they would already be prepared for both model A and model D.

Is the student able to start working mathematics in Spanish until the 8th course? Will it not explain delay with those who have learned Basque?

PaP: But when that step is taken, what is the most inconvenient: linguistic changes or the difficulties of the subject?

J. F. F. L.:

Both are important and both are united. Anyone could think that it would be nothing more than a lack of custom, a lack of habit that would be overcome after a few bad months. In part it is like this, but not just that.

In the Teaching Media, concepts and contents are complicated by demanding a greater linguistic development. Models D or A may also have this problem, but the gap that others may have is more serious. We believe that although when analyzing the conclusions we will make more concrete, linguistic change should be made before moving to the CPR.

PaP: How have you proposed empirical study?

J. F. F. L.:

So far I have tried to account for the theoretical approach, but this work has not been a mere theorization. When designing the study we thought we had to make four different groups of 8th course:

Group formed by a number of students of model A (group A) with students of model B, two groups (group B and group B) another group of model D (group D)

A and B passed the tests in Spanish and D and B’ in Basque. Since our study was aimed at model B, here we had the opportunity to compare it with two groups in Basque and Spanish: Group B with A and B’ with D.

To complete the sample, we went to the public schools and the ikastolas of the Basque Autonomous Community with the aim of getting about 900 students. We did not go to private centers, among other things because in Alava we did not find at least D.

The variables considered would be, on the one hand, the mathematics itself as dependent variable (distinguishing the calculation to be addressed within mathematics, the understanding of an explanation, the understanding and application of the written text) and, on the other, the level of Basque that we have considered as independent variables, the type of center, the linguistic model, the socioeconomic level, the age, the sex, etc.

PaP: How have you measured the level of mathematics?

J. F. F. L.:

We used three different tests to measure mathematical knowledge:

1. Foreign test EIA ( International Evaluation Achievement ) of the United States. Starting from the Spanish version, before passing to the students, we had to translate, validate and control the reliability. This was the first step. This test consists of 40 items that collect contents of level 8: geometry, fractions, arithmetic,... With this test four different processes are measured: calculation, understanding, application and analysis (those related to the analysis are only two items and having no significant influence on the research have hardly been taken into account).
2. In order to measure the understanding of the teacher's exposure, a CPR professor, after passing an explanation previously recorded in video, we asked them about it. The length of the video is an hour and a half and, of course, it has had two versions, one in Basque and one in Spanish.
3. To measure the understanding of the written text, the topic of the probability of the book BBB-1 published by Elhuyar was selected. Not complete, it is too long. Since the text was in Basque, it was translated into Spanish to move to groups A and B. After reading the text, we had to answer several questions, some in Basque and others in Spanish.

Both when choosing the probability test and when choosing the video test, we have limited two main conditions: on the one hand, the fact that the subject is new, since if the results were previously treated, they would be “not real”, and on the other hand, the fact that it is a subject at the level of development of the student.

To measure independent variables, we had to create some tests, but not all, such as the standardized D-48 test that we used to measure intelligence. The proof we have created is the adaptation to the story Peru makur. In this test in which, after reading the story, the corresponding questions appear, your reading level in Basque will be measured. Three aspects are mainly measured: reading speed, percentage of understanding and percentage of reading speed.

The new tool involves the realization of an “extra” work prior to its incorporation into real research, such as the realization of the same test and the control of reliability and utility. Once this had been done, he would be willing to pass it.

PaP: Are the measuring tools used by you usable by any teacher?

J. F. F. L.:

In general yes, although some tests have been specially prepared for this research (Video test, text used to measure probabilities and test based on the story Peru makur). The rest, however, is accessible to anyone, as long as the level of the students is 8 or higher (test EIA, test D-48 and story Peru makur).

PaP: What would be the most remarkable conclusion?

J. F. F. L.:

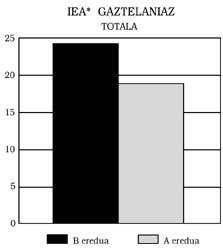
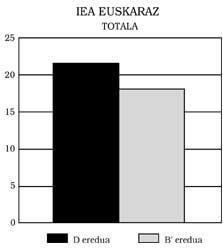
Three main conclusions can be drawn: 1) The students of model B present a minimum level equivalent to those of model A in Spanish. This means: Those of model B show no mathematical delay, although the teaching model is bilingual. Moreover, as can be seen in Figures 1, 2, 3 and 4, they have obtained better scores than those of model A. In some cases, moreover, the differences have been statistically significant, despite the control of intelligence and socioeconomic level. Anyone might think that the best score is promoted by the beneficial effect of bilingualism, but we have not dared to say so. A deeper investigation, which has not been one of our goals.

The real reason for this difference between models A and B, as we have analyzed, would be in the type of center. While in model B are the ikastolas and public centers, in model A only public centers (in the ikastolas there is no model A). On the other hand, the context in which the public centers of model A are located is usually marginal, in which there are abundant and frequent problems of integration, adaptation, etc.

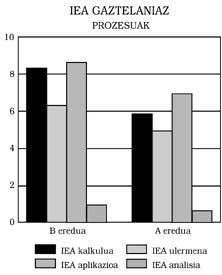
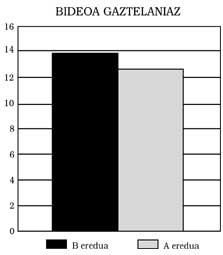
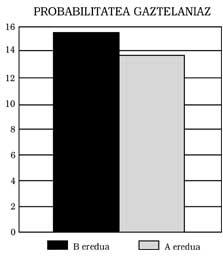
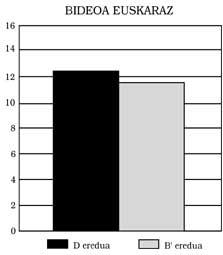
This can be the main reason for this difference. 2) Comparing those of model B’y D, in all the tests the D’s were shown above (see figures 5, 6, 7 and 8 for this). These differences are significant in aspects such as the application and explanation of a teacher or the understanding of a text, but in others such as the calculation does not occur. What is your explanation? Having a higher linguistic level, in those cases where the influence of the language is high, those of model D obtain better results. 3) The third conclusion is that the results obtained in other bilingual contexts have been contrasted, that is, that the more the second language has been worked and used in school, the greater the degree of competence, without the first language suffering any delay.

What has happened in our case? We have observed significant differences between models B. In any case, when we talk about the model B, it must be said that it is a very generalized model, since models B plus euskaldunes and castellanoparlantes can be found. This classification is done according to three variables: percentage of euskaldunes between teachers, percentage of subjects of the higher cycle that are studied in Basque, and finally, from course 6, work mathematics in Basque or in Spanish.

The difference between models B is real, since in mathematical tests the model B plus euskaldun gets a better score. When passing the test in Spanish there have been hardly any differences. In short, what is achieved with language in some bilingual contexts, we have achieved with mathematics, that is, the more we work mathematics in the second language, the more competition is in the second language without delays in the mother tongue.

The truth is that these results have not made us believe too many unforeseen events, perhaps the biggest of the unforeseen events was the difference between models B and A, which we expected inverse results. We have said, however, what your reason may be.

PaP: How would you restructure teaching to these conclusions so that the results are ‘optimal’?

J. F. F. L.:

According to the above, the structure of mathematics in model B would first be modified, at least in terms of the use of the language (if one wants to reach the main objective to which reference has been made, that once the level 8 is finished, he will be able to study his studies in both Basque and Spanish). In order to do this, it is necessary that from a certain level the mathematics in Basque is started to work, and as we say in the pedagogical implications of the thesis, in the current system level 6 would be the most appropriate to take that step.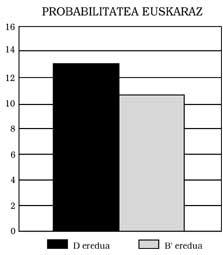

However, with the next reform, we do not know how much or how things are going to change, although bear in mind that this is only one of the possible ways, which has seemed more appropriate to us, but each center is going to mark its way. Why choose 6th and not any other? On the one hand, because the level of Basque of students of 6th is quite rich and, on the other, because by distinguishing the Basque language from the limits of Spanish, there is no interference. How to carry out this teaching? There are no magic formulae, either giving some subjects in Basque and others in Spanish, or treating each topic in two languages (introduction and review in one language and the rest), or in any other way.

However, and in the pedagogical implications we have tried to clarify, when it comes to starting to work mathematics in the second language, aspects such as the elaboration of a specialized vocabulary, the treatment of problems, the development of the first and the second language, etc. There is, therefore, something elaborate and what to arrive if we want to become truly bilingual.