Renaissance Physics
The research of the field currently occupied by Physics had a time of transition in the Renaissance. Modern physics XVII. It did not materialize until the mid-twentieth century, when the mathematical tool was used in the expression and research of the phenomena of the physical world. Mathematics and astronomy XV. and XVI. Although in the centuries there were basic changes (in the first of them from the hand of abstraction in the approach of problems and in the second of the Copernican system), in Physics there was no such change.

If we look at the previous way to understand this, that is, in the last century, the reason for this difference clearly appears. Astronomy and Mathematics knew two works of great importance that served as an example for the next centuries in the classical era, the Elements of Euclid and the Almagest of Ptolemy. However, in the field of classical physics this type of work was not known. The most outstanding work of Greek physics is that of Archimedes (both in capacity and on the path that later this science would follow), but he had not at all the body of the two works cited, nor his roundness and generality. Also, we must say: Archimedes' physics, quite initiated, was almost unknown.
In the field of physics, as in other thousand fields, from the Greek era to the sixteenth century. Until the 20th century the reference point was Aristotle. That physics was totally qualitative and in it (predominantly mechanical problems) mathematics did not take place. Therefore, knowing that the era of modern physics began with the mathematical treatment of movement and its causes should not surprise us.
The University of Paris’ Low-Medieval nominalist school made a profound critique of the theory of the Aristotelian movement. The answer to these problems remained in the impetus theory. On the other hand, the nominalists and earlier some scholastics (albeit very modestly) introduced the world of physics, somehow, the mathematical tool. In Aristotle quantity and quality were two totally different categories and no relationship could be established between them. For these medieval thinkers quality was something that could be measured numerically, that is, they thought that a gradation could be done as what is done with qualities, with quantities.
Oxford tradition
The concern of scholastics was never the resolution of special scientific problems. On the contrary, if they ever addressed any scientific problem, they included it in a general philosophical framework, usually referred to one example and not another. His responsibility was above all the methodological part. However, XIV. Throughout the 20th century new approaches to the research of nature phenomena were published, which would later prove prolific in the creation of modern science. As an example of all this, we have works by Grosseteste, Witelo or Theodoric by Freideberg. These and others tried to somehow use mathematics to investigate the movement.

As a starting point is what today we would say a functional relationship, that is, a systematic consideration between causes and effects. From this point of view, the expression of any phenomenon could be given through an algebraic function, provided that all the necessary conditions were known, that is, the relationship between independent and dependent variables is claimed. The formulation of these functional relationships was carried out by two methods. The first is the word algebra. This was used by Brawardino in his writings on mechanics, in which he used letters of the alphabet instead of variable amounts to obtain generality, but mathematical operations (addition, multiplication, etc.) needed expressive words. This method achieved great success in Oxford.
Another way of expressing functional relationships was that of the geometric way. XIV. At the beginning of the 20th century, both in Oxford and in Paris, the expression of the intensive of a quality was widespread by direct coordinates. One of the inventors of this path was the Joan de Dumbleton. In his book Summa logicae et philosophie naturalis, he made all the critical expression of the physics of his time.
XIV. Throughout the 20th century, scientists tried to quantitatively express qualitative changes and this had its consequences in the field of mathematics and physics. For example, for Ockam the only way to objectify time was by representing all consecutive positions of a body with uniform motion, and based on it, they could then comparatively offer the duration of movement of any other movement. Among the doctors they knew a certain numerical scale to express cold and heat. The widespread one divided the climbing of cold and heat into groups.
All these considerations are of great importance, since the Aristotelian physics in force at the time was sifted and, on the other hand, it is necessary to take into account the poor condition of the meters that could be used: the water and sand clocks (later mechanical; the pendulum clock would not be invented until 1657), the instruments used by the merchants to measure squads, compasses, balances, length, volume and weights, and astronomical.

The method of measurement and experimentation went from Oxford and Paris to Germany and Italy. One of the most enthusiastic defenders of this method was Nicola de Cusa, who tells us about the use of the balance to know the proportion of the first elements within metals and spirits (mercury, salt, sulfur, ammonia and arsenic). He also proposed as a gauge the balance to express the virtue of the magnet. Invented a hygrometer to measure air weight, etc.
However, the tests of quantification of the qualities performed by scholastics are basically different from those of modern science. On the one hand, in his theoretical works no real data appear, that is, the application of mathematics does not come from observation, but from elubration. On the other hand, the tendency to their generality led them to worry about logic above all else and, incidentally, to make more absurd extrapolations. Therefore, the fact that all this is considered a pioneer of modern science has its problem. However, the influence of this methodology on the subsequent epistemological rupture is not negable.
School Impetusa
This theory appeared and spread among Oxford thinkers, but its full and developed formulation was later obtained in Paris and finally the XVI. Until the end of the 20th century it took its place in all European universities. It must be said that the Leonardo da Vinci, for example, which has been used as an archetype of Renaissance science, was a slave to this theory and that on other occasions, when historians have considered this man's special contributions to the theory of movement, it has been seen that, in most cases, they are adaptations of Parisian scholastics. On the other hand, it is clear that the work on the Galileo youth movement is related to this school.
The scholastics found above all in their Aristotelian thesis of movement two difficulties: the movement of shells and heavy pieces. For Aristotle and his followers, unlike what is today due to inertia, the cause was movement and not inactivity. Therefore, for a body to have movement it must have a motive cause in physical contact and without interruptions. The interruption of this driving cause will lead to the cancellation of the movement. On the other hand, they admitted that if the obstacle that counterposes the mobile the interval persists constant, its speed would depend on the force imposed on it. Therefore, how can we express the acceleration observed in the fall of weight parts? Why does the arrow not fall to the ground as soon as it loses contact with the bow cord? Between theory and what was seen there was a big hole.

Aristotle's followers somehow expressed these discrepancies. For example, to express the continuous movement of the projectiles the change produced by the initial thrust into the air was accepted, that is, as the air preceding the end was driven and compressed, the projectile was swiftly moved backwards and pulsed so that no vacuum in the air would occur (something unacceptable to them). Both Leonardo da Vinci and his guards of the time admitted this cult. When expressing the movement of the pieces that weigh, and seeing that it is an accelerated movement, they used another argument based on air, that is, the closer the surface body is, the greater the weight of the atmosphere on it and the less the obstacle of the air column below it. Therefore, the body accelerates.
The most profound criticism of this theory of movement (there were others before and after) was the XIV. They were created in Oxford and Paris in the 19th century. This is that of Joan Buridan, rector of the University of Paris twice. In two books, Quaestiones super octo libros physicorum and Quaestiones de coelo et mundo, he captured his theory. This theory starts from the need for an engine for movement, so it can somehow be considered as the final development of Aristotelian theory.
The impetus was a peculiarity that the bodies received for being in motion. The impetus in the projectiles is constantly decreasing, both by the discomfort of the air and by the gravity towards the ground. In the case of weight pieces, the impetus was something like gravity overlapped by motion. Thus, its acceleration was a consequence of the sum of consecutive impetus. They considered that the impetus of any body was the product of its quantity of materi for its speed.
Among other things, the merit of this theory is to express itself through a single grip or ground dynamic.

This path of analysis had its reflection in the Renaissance. Nicola de Cusa, for example, appears as his follower and Leonardo da Vinci or Kopernik. Cardano, in his work Of subtility (1550), not entirely, accepts it in part.
Science and Technology
Throughout the medieval centuries the solid foundations of technological change were established, but the economic, philosophical or social environment was not the most suitable for its materialization. Both the growth of trade and the development of the bourgeoisie would be the engine of this corpus and the creation of the modern world. B. As Gille said: XV. At the end of the 20th century it became technical one of the main activities of modern society. Of course, the relations between technology and science were scarce.
However, the environment was changing. XIII. In the 20th century Roger Bacon wrote: If I could, I would burn all of Aristotle's books. To study is not to waste time, but to lead to error and to increase ignorance. And later: remove yourself from the hands of the dogmas and the mandate of the authorities: look at the world. It may be thought that in his day this revolutionary message had no success. However, it indicates the trend of a current. In the quattrocento, for example, the unacceptable of the last century would find a much more suitable environment and to understand it must take into account the social changes that have occurred, including the conjugation between science and technology.
In classical Greece there was enormous opposition between the liberal arts (i.e., those practiced by free men) and the mechanical arts (i.e. those used by slaves). This attitude lasted during the Middle Ages. While theorists (i.e., philosophers or theologians or university professors) enjoyed great prestige and prestige, practitioners (craftsmen or engineers or architects, that is, those who use their hands or have their works directly related to production) are at a much lower level, although they were not received with contempt.

This situation underwent a profound change in the Renaissance. Mining technicians, architects, watchmakers and all craftsmen were welcomed with respect for the power they had to dominate nature. Therefore, mechanical arts came to the fore in tune with theoretical works. In this sense, some theorists tried to defend or exalt the mechanical arts and others to fight against the mere theoretical situation. Bernard Palissy, for example, known Parisian ceramist, published in 1580 his admirable Discours, where you can find a thousand reasons contrary to the culture that was then taught in the Sorbonne.
He claimed that true philosophy is only the art of the study of nature, that is, something that all (and not only philosophers or theologians) can do. As Palissy we can cite Rabelais, Luis Vives or Andrea Vesalius of the same century. All of them, without excluding theoretical studies (but the other way around), chose technical activities, that is, positive knowledge versus mere speculation or literary practice, rejecting somehow the concept of science of Aristotle. From then on the wedding that took place in that century would bear fruit until now.
Movement and static
XVI. Due to the development of the firearms industry in the 18th century, the old problem of shells reappeared, but this time raised from a practical perspective. As their small weapons improved, the manufacture of large caliber cannons began in Germany. The small arms had no theoretical problems, but as the big ones had greater power, they produced problems of aim. In 1537 Tartaglia published the book Nova sciencia, a new science that was nothing more than a ballistics. Through this book he intended to adapt the theoretical expression to what was still an empirical knowledge.
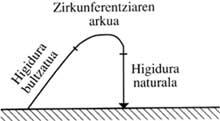
Aside from philosophy (which he wrote the book for use by technicians), he addresses work through mathematics. Tartaglia takes a euclidean path: in the beginning it puts some axioms and some assumptions and from them it draws some conclusions for the dynamic investigation of the projectiles. However, the mechanics it uses are the usual. Therefore, movement can be natural or forced and free fall is the only existing natural movement. In the natural movement the speed of the mobile increases as it moves away from its origin or approaches its destination. The exact opposite happens in the forced movement. The intrinsic difference between these two types of movement makes it impossible for them to occur simultaneously. Therefore, the natural movement starts immediately after the push and is tangent to it. Although it does not correspond with the said and with the observations, drawing the trajectory of the projectiles shows three phases: two straight paths joined by an arc of the circumference.
Almost ten years later he wrote another book: Onestiti et inventione. In it, when it analyzes the movement of the projectiles, when it is not vertical, it accepts as a curve the path of all the driven movements. However, this point of great importance was not taken with attention.
Apart from the movement, in 1586 we found Static. That same year Simon Stevin wrote about static in Dutch his book De beghinselen der weeghconst on applications and a little later another on applications and then the third on hydrostatic.
Static is divided into two books. It investigates the properties of balance between weights and centers of gravity of flat and solid images. At first, after giving some definitions and postulates, investigate the case of certain weights (to understand the lever) that pull vertically. It then explores the case of inclined forces by introducing the inclined plane. From the investigation of a set of weights, joined by a cord and hung from a triangular prism, came the idea of a parallelogram of force, which was later used in complex machines.

In the field of hydrostatics, Stevin gave us his first achievements since the time of Archimedes. After checking that the liquids are in balance, regardless of their diameter, in the containers communicated, he verified that the pressure on the bottom is only related to the height of the liquid. He would then take care of the pressure of the side walls, including the case of curved walls.
Buletina
Bidali zure helbide elektronikoa eta jaso asteroko buletina zure sarrera-ontzian


