The great temple of Benares

1992/10/01 Angulo, Patxi Iturria: Elhuyar aldizkaria

• Matematika

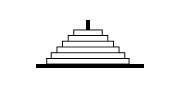
The sacred city of Benares is located at the mouth of the river Ganges. According to legend, in the great temple of Benares, under the dome that represents the center of the world, is a silver sole with three diamond needles. In creation, the god put 64 discs of different diameter on a needle, from the largest on the tray to the smallest on the tip of the stack. Thus the tower of Brahman is formed. The priests change the discs from one needle to another constantly day and night, according to the fixed and immutable rules of Brahman, that is, the discs must be changed one by one and one cannot be placed on a smaller one. The day the 64 discs change from the needle that the gods put in creating the world to one of the other two, that day the tower, the temple and the brahmandarras will become dust and with a great dunbots everyone will disappear.

This old legend poses a problem: to calculate the day when the world has to disappear. For this we must find the least number of movements necessary to make the change. However, in addition to limiting this day, this problem poses another aspect that we consider more interesting: the calculation method. In fact, the legend of the temple of Benares is a good example of research. This game, in addition to its motivating character, is an example of a problem that adapts to the different learning rhythms of the students, being able to carry slow and fast rhythms at the same time.

There is no need to go to Benares to play this kind of games. Three wedges are placed on a tablet and only wood discs of different sizes are needed, or three circles are represented on a sheet and coins of different sizes are obtained.

Didactic suggestions

In order for students to take good ownership of the game and the rule, they will speak with less coins or discs. In this first stage, the rules will be given priority and not the number of movements.

The second phase will consist of two, three, four, five discs. They will count the number of movements needed to change pallet in each case. The results obtained by each student will be compared with those obtained by the rest.

Number of disks 123456Minimum movement number1371531...Table 1.

In the third phase you will be instructed to make the change with the least possible movement and repeat the exercises of the previous phase. They will compare the results again and save them in a table.

The next step will warn students that it is not possible to use the experimental method to calculate the least number of movements when the number of disks increases. For example, they may be asked to modify the seven-disc tower. Given the difficulty, students should be encouraged to look for the mathematical formula or model that gives us the least number of movements for any number of albums.

They will be asked to analyze the relationship between the discs and the number of movements, as well as the relationship between the number of movements. The answers, proposals, ideas,... If necessary, a series of suggestions will be made: difference between consecutive quantities, how to obtain an amount knowing the previous one,...

1 = 2 to 1

3 = 2 x 2 - 1

7 = 2 x 2 x 2 - 1

15 = 2 x 2 x 2 x 2 -1

31 = 2 x 2 x 2 x 2 x 2 x 2 x 2 - 1

By:

Following this path they will obtain formulas for the first cases before late (Table 3). From there we will give the general formula, completing in some cases the calculations.

Number of discs 12345678910Number of movements 137153163txika2 1 - 12 2 - 12 3 - 12 4 -12 5 - 12 6 - 1

Returning to the legend, the lowest number of movements needed to change the 64 discs per needle, according to the formula obtained, is 2 64 – 1. Once the calculation is done,

18 3 446,744 2 073,709 1 551,615

We get the number. Literally: eighteen trillion, four hundred forty thousand, seven hundred forty-three trillion, sixty-three thousand, seven hundred nine million, five hundred fifty-five thousand, six hundred and fifteen (that is the number of galgas that appears in the chess legend).

Assuming they need a second to make each move, and acting constantly, they would need 584,942 1 417,200 years, approximately, to carry out all the change. Given that the Earth is 3,000 million years old, we cannot say that we are in danger.

Based on the above, we can invent other towers that limit the duration of the world. They follow the same fixed and unalterable standards.

Tower of Xiva-Vixun

The discs of the Brahman tower are numbered from minor to major. Odd on left needle

and the pairs are on the right. The goal is to leave the pairs to the left and the odd ones to the right (start with few discs).

Tower of Allah-Jainko-Kung Ts

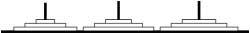
The number of disks must be multiple of three. They are inserted into three needles. The goal on this occasion is to pass the discs from left to center, those from center to right and those from right to left.

Tower of Atheists

The discs are distributed randomly on the needles (one, two or three), in this distribution the discs

can remain in any order. With always fixed and immutable standards, all discs must be sorted on a needle.